Relative-Motion Analysis: Acceleration

Learning Goal:

To be able to use the equation for relative acceleration between two points to find unknown linear accelerations and angular accelerations of connected bodies.

Member ABC is controlled using a rack and pinion, which is connected at B. (The rack is the long, horizontal gear.) The rack can only move horizontally and the pinion does not translate. A block in a sleeve is connected at A and allows movement only in the vertical direction. Member ABC has a total length of

2.30 m and the distance between A and B is 1.15 m . When member ABC forms an angle of θ=31.0∘ with the rack, the acceleration of B is aB=1.55 m/s2 and the velocity of the block attached at A is vA=2.45 m/s in the direction shown. Positive angular velocity and acceleration are in the counterclockwise direction.

 

 

 

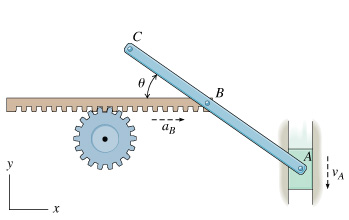
Part A - Acceleration of A

Find the acceleration of A at the instant shown. Indicate the direction with the sign based on the axes in the figure.

Express your answer to three significant figures in meters per second squared.

aA=

11.2

j m/s2

 

 

Part B - Acceleration of C

Find acceleration of C, aC, at the instant shown. Give your answer in component form.

Express your two answers, separated by commas, to three significant figures in meters per second squared.

aC=

3.10,-11.2

i, j m/s2

 

 

Part C - Acceleration of B‎

Find the acceleration of B when the acceleration of C is aC=4.15 i+3.00 j m/s2 and the acceleration of A is aA=−3.00 j m/s2 . Member ABC makes an angle of θ=19.0∘ at the instant considered.

Express your answer to three significant figures in meters per second squared.

aB=

2.08

i m/s2