± The Work of a Force
Learning Goal:
To be able to calculate the work done by a force.
If an external force, F , acts on a rigid body, the work, UF , done by the force when it moves along a path s is defined as
UF=∫rF⋅dr=∫sFcosθds
where θ is the angle between the force and the object's direction of motion and r is the position vector that points to the object as it moves along path s .
Part A
Which of the following forces do work?
- A compressed spring that is allowed to expand.
Part B
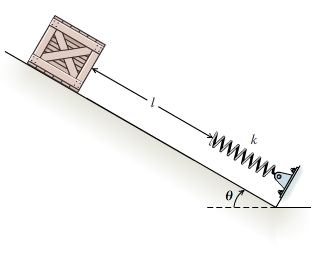
A crate of mass m = 54.0 kg slides a distance l = 4.60 m down a ramp at an angle θ = 25.0 ∘ . At the bottom of the ramp is a spring that has a spring constant k = 680 N/m . (Figure 1) What is s , the distance the spring will compress when the crate comes to rest? The ramp is smooth enough that the friction is negligible.
|
|
2.10
|
|
Part C
When
a satellite is launched, its orbital radius is usually determined far
in advance because the amount of energy required to change its orbit is
large. How much work, W , would a rocket thruster on a satellite of mass m = 610 kg need to do to change the satellite's orbital radius from r1 = 2.84×107 m to r2 = 4.16×107 m ? (Figure 2) The Earth's radius is RE = 6.37×106 m . Assume that both orbits are circular.
|
|
2.71×109
|
|